Difference between revisions of "Minimal negation operator"
Jon Awbrey (talk | contribs) (typos) |
Jon Awbrey (talk | contribs) |
||
| Line 228: | Line 228: | ||
==Charts and graphs== | ==Charts and graphs== | ||
| − | This Section focuses on visual representations of minimal negation operators. A few | + | This Section focuses on visual representations of minimal negation operators. A few bits of terminology are useful in describing the pictures, but the formal details are tedious reading, and may be familiar to many readers, so the full definitions of the terms marked in ''italics'' are relegated to a Glossary at the end of the article. |
Two ways of visualizing the space <math>\mathbb{B}^k</math> of <math>2^k\!</math> points are the [[hypercube]] picture and the [[venn diagram]] picture. The hypercube picture associates each point of <math>\mathbb{B}^k</math> with a unique point of the <math>k\!</math>-dimensional hypercube. The venn diagram picture associates each point of <math>\mathbb{B}^k</math> with a unique "cell" of the venn diagram on <math>k\!</math> "circles". | Two ways of visualizing the space <math>\mathbb{B}^k</math> of <math>2^k\!</math> points are the [[hypercube]] picture and the [[venn diagram]] picture. The hypercube picture associates each point of <math>\mathbb{B}^k</math> with a unique point of the <math>k\!</math>-dimensional hypercube. The venn diagram picture associates each point of <math>\mathbb{B}^k</math> with a unique "cell" of the venn diagram on <math>k\!</math> "circles". | ||
Revision as of 13:28, 24 August 2009
The minimal negation operator \(\nu\!\) is a multigrade operator \((\nu_k)_{k \in \mathbb{N}}\) where each \(\nu_k\!\) is a \(k\!\)-ary boolean function defined in such a way that \(\nu_k (x_1, \ldots , x_k) = 1\) in just those cases where exactly one of the arguments \(x_j\!\) is \(0.\!\)
In contexts where the initial letter \(\nu\!\) is understood, the minimal negation operators can be indicated by argument lists in parentheses. In the following text a distinctive typeface will be used for logical expressions based on minimal negation operators, for example, \(\texttt{(x, y, z)}\) = \(\nu (x, y, z).\!\)
The first four members of this family of operators are shown below, with paraphrases in a couple of other notations, where tildes and primes, respectively, indicate logical negation.
|
\(\begin{matrix} \texttt{()} & = & \nu_0 & = & 0 & = & \operatorname{false} \'"`UNIQ-MathJax1-QINU`"' * The point \((0, 0, \ldots , 0, 0)\) with all 0's as coordinates is the point where the conjunction of all negated variables evaluates to \(1,\!\) namely, the point where:
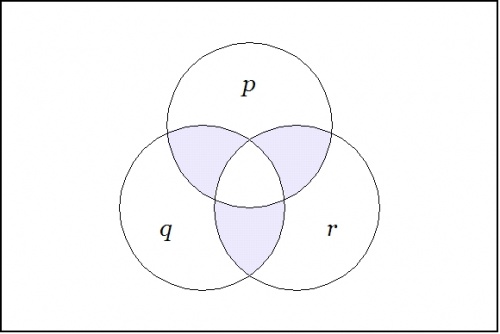
To pass from these limiting examples to the general case, observe that a singular proposition \(s : \mathbb{B}^k \to \mathbb{B}\) can be given canonical expression as a conjunction of literals, \(s = e_1 e_2 \ldots e_{k-1} e_k\). Then the proposition \(\nu (e_1, e_2, \ldots, e_{k-1}, e_k)\) is \(1\!\) on the points adjacent to the point where \(s\!\) is \(1,\!\) and 0 everywhere else on the cube. For example, consider the case where \(k = 3.\!\) Then the minimal negation operation \(\nu (p, q, r)\!\) — written more simply as \(\texttt{(p, q, r)}\) — has the following venn diagram:
For a contrasting example, the boolean function expressed by the form \(\texttt{((p),(q),(r))}\) has the following venn diagram:
Glossary of basic terms
See alsoTemplate:Col-break
External links
|